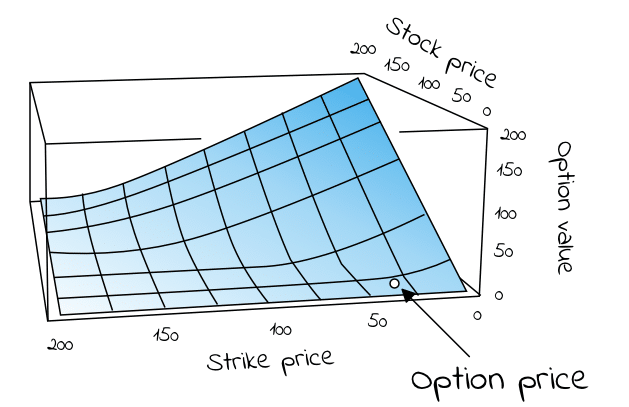
MarketXLS offers you simple commands to fetch historical and live option prices, but have you wondered how options contracts are priced?
Any option’s premium consists of two components: the contract’s intrinsic and extrinsic value. The intrinsic value signifies the difference between the strike price of an option and the current market price of the underlying instrument. For a call option, the intrinsic value is the current price – the strike price, and for a put option, it is the strike price – the current price.
The extrinsic value consists of several factors such as time to expiration, implied volatility, interest rates, and dividend yields, among others. In other words, any premium over intrinsic value is said to form the extrinsic value. The premium is what an investor is willing to pay above the intrinsic value in the hope that the value of the contract increases due to changing market conditions.
Because of the involvement of several factors in the option premium, calculating the premium is a challenging task and is accomplished with the help of mathematical models. Several models are used in practice, like the Black-Scholes model, Heston Model, Morton Framework, etc. However, one of the most commonly used models is the Black-Scholes model.
The Black Scholes Model –
The Black Scholes model assumes no-arbitrage pricing for the options contracts and uses five key inputs to derive the option price. These inputs are namely:
- Strike price,
- Current price of underlying,
- Interest Rate,
- Implied Volatility, and
- Time to expiry
For a Call option, the option price is derived using.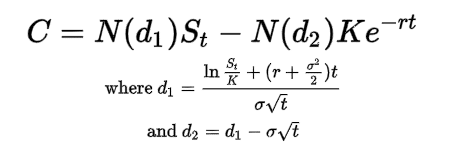 C = call option price
C = call option price
N = normal distribution
St = Current price of the asset
K = strike price
r = risk-free interest rate
t = time to maturity
𝜎 = implied volatility of the asset
Fortunately, you don’t have to do this calculation yourself. Instead, MarketXLS give you predefined functions that you can directly run in excel to get the output.
But does it mean that actual market prices are always equal to the value derived from the BSM? Sadly, No. The BSM model is based on certain assumptions which may or may not hold in the real world, leading to a mismatch between the values derived from the BSM and market values.
These assumptions are as follows:
- Short term interest rates and volatility are constant
- There are no transaction costs associated with buying or selling options
- The options in consideration are European
- The returns of the underlying stock are normally distributed
- The markets are perfectly liquid
The Bottom Line –
Due to the impractical nature of these assumptions, market participants often notice huge differences between the values derived from BSM and actual market values. These differences are often driven by changing interest rate and volatility assumptions and supply and demand equations. However, the value derived from the BSM model can be used as a starting point while analyzing options.